Return the root node of a binary search tree that matches the given preorder traversal.

(Recall that a binary search tree is a binary tree where for every node, any descendant of node.left has a value < node.val, and any descendant of node.right has a value > node.val. Also recall that a preorder traversal displays the value of the node first, then traverses node.left, then traverses node.right.)

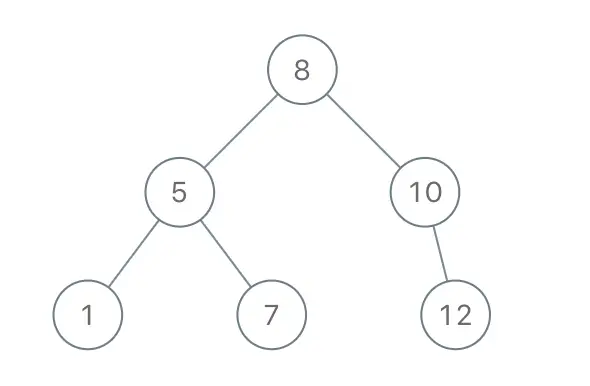
Input: [8,5,1,7,10,12]

Binary Search Tree

Output: [8,5,10,1,7,null,12]

Note:
1 <= preorder.length <= 100
The values of preorder are distinct.

Divide and Conquer (Recursion) in Python

The root is the first element of the list. Then, we can find the sublist which has the elements smaller than the root, and the sublist with the elements larger than the root. Therefore, we can recursively construct the binary search tree using the divide-and-conquer technique.

The following Python recursion implementation is using the list list comprehension. You might also search the element and construct the list using the array slicing or simply passing the left and right index into the recursion.

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17

# Definition for a binary tree node.
# class TreeNode:
#     def __init__(self, x):
#         self.val = x
#         self.left = None
#         self.right = None
 
class Solution:
    def bstFromPreorder(self, preorder: List[int]) -> TreeNode:
        if len(preorder) == 0:
            return None
        root = TreeNode(preorder[0])
        smaller = [x for x in preorder[1:] if x < preorder[0]]
        larger = [x for x in preorder[1:] if x > preorder[0]]
        root.left = self.bstFromPreorder(smaller)
        root.right = self.bstFromPreorder(larger)
        return root

# Definition for a binary tree node.
# class TreeNode:
#     def __init__(self, x):
#         self.val = x
#         self.left = None
#         self.right = None

class Solution:
    def bstFromPreorder(self, preorder: List[int]) -> TreeNode:
        if len(preorder) == 0:
            return None
        root = TreeNode(preorder[0])
        smaller = [x for x in preorder[1:] if x < preorder[0]]
        larger = [x for x in preorder[1:] if x > preorder[0]]
        root.left = self.bstFromPreorder(smaller)
        root.right = self.bstFromPreorder(larger)
        return root

The Java and C++ implementation of the same algorithm can be found here: How to Construct Binary Search Tree from Preorder Traversal? (C++ and Java)

Related Binary Tree Construction Algorithms

You may also like the following posts on the similar tree problems.

• Recursive Algorithm to Construct Binary Tree from Preorder and Postorder Traversal
• How to Construct Binary Search Tree from Preorder Traversal in Python?
• Algorithm to Construct Binary Tree from Preorder and Inorder Traversal
• How to Construct Binary Search Tree from Preorder Traversal? (C++ and Java)
• How to Construct String from Binary Tree?
• How to Balance a Binary Search Tree using Recursive Inorder Traversal Algorithm?
• How to Construct the Maximum Binary Tree using Divide-and-Conquer Recursion Algorithm?
• How to Construct Binary Tree from Inorder and Postorder Traversal using Depth First Search Algorithm (Recursion)?
• How to Construct Binary Tree from String (Binary Tree Deserialization Algorithm)

–EOF (The Ultimate Computing & Technology Blog) —