Depth First Search Algorithm to Compute the Smallest String Star

  • 时间：2020-09-09 13:16:32
  • 分类：网络文摘
  • 阅读：92 次

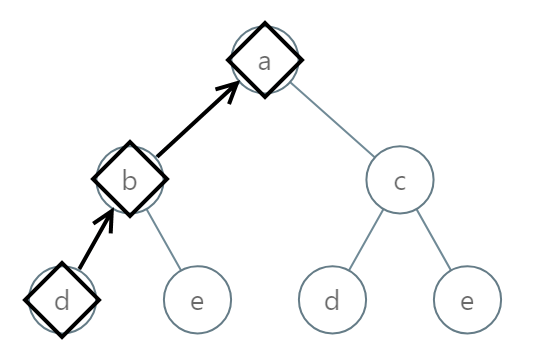
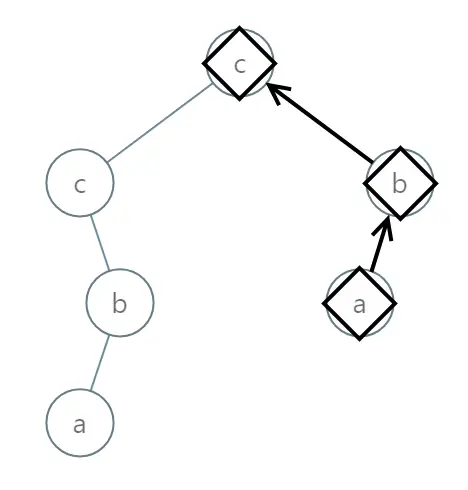
Given the root of a binary tree, each node has a value from 0 to 25 representing the letters ‘a’ to ‘z’: a value of 0 represents ‘a’, a value of 1 represents ‘b’, and so on.

Find the lexicographically smallest string that starts at a leaf of this tree and ends at the root.

(As a reminder, any shorter prefix of a string is lexicographically smaller: for example, “ab” is lexicographically smaller than “aba”. A leaf of a node is a node that has no children.)

Example 1:
Input: [0,1,2,3,4,3,4]
Output: “dba”
binary-tree1 Depth First Search Algorithm to Compute the Smallest String Starting From Leaf algorithms c / c++ DFS recursive

Example 2:
Input: [25,1,3,1,3,0,2]
Output: “adz”
binary-tree2 Depth First Search Algorithm to Compute the Smallest String Starting From Leaf algorithms c / c++ DFS recursive

Example 3:
Input: [2,2,1,null,1,0,null,0]
Output: “abc”
binary-tree3 Depth First Search Algorithm to Compute the Smallest String Starting From Leaf algorithms c / c++ DFS recursive

Note:
The number of nodes in the given tree will be between 1 and 8500.
Each node in the tree will have a value between 0 and 25.

Recursive Depth First Search to Compute the Smallest String Starting From Leaf

We can start from the Root, perform a recursive DFS (Depth First Search) algorithm, and pass the string along down to the leaves. When we reach a leaf node, we construct the final string, and store the smallest one (alphabetically).

All nodes are visisted exactly once. When we finish searching the tree, we have the answer. The time complexity is O(N). In this particular case, the DFS is also the bruteforce algorithm.

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    string smallestFromLeaf(TreeNode* root) {
        dfs(root, "");
        return ans;
    }
private:
    string ans = "";
    void dfs(TreeNode* root, string cur) {
        if (!root) return;
        if ((root->left == nullptr) && (root->right == nullptr)) {
            cur = (char)(97 + root->val) + cur;
            if ((ans == "") || (cur < ans)) {
               ans = cur; 
            }
            return;
        }
        dfs(root->left, (char)(97 + root->val) + cur);
        dfs(root->right,(char)(97 + root->val) + cur);
    }
};
/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    string smallestFromLeaf(TreeNode* root) {
        dfs(root, "");
        return ans;
    }
private:
    string ans = "";
    void dfs(TreeNode* root, string cur) {
        if (!root) return;
        if ((root->left == nullptr) && (root->right == nullptr)) {
            cur = (char)(97 + root->val) + cur;
            if ((ans == "") || (cur < ans)) {
               ans = cur; 
            }
            return;
        }
        dfs(root->left, (char)(97 + root->val) + cur);
        dfs(root->right,(char)(97 + root->val) + cur);
    }
};

Depth First Searching Algorithm using Stack

The Recursion uses implicit stacks. The compiler generates the calling stacks. The recursion code usually is more clean and concise. We can also use a stack to manually emulate the recursive calls.

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    string smallestFromLeaf(TreeNode* root) {
        if (!root) return "";
        stack<pair<TreeNode*, string>> st;
        st.push({root, ""});
        string ans = "";
        while (!st.empty()) {
            auto p = st.top();
            st.pop();
            if (p.first->right) {
                st.push({p.first->right, (char)(97 + p.first->val) + p.second});
            }            
            if (p.first->left) {
                st.push({p.first->left, (char)(97 + p.first->val) + p.second});
            }
            if ((p.first->left == nullptr) && (p.first->right == nullptr)) {
                string v = (char)(97 + p.first->val) + p.second;
                if ((ans == "") || (v < ans)) {
                    ans = v;   
                }                
            }
        }
        return ans;
    }
};
/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    string smallestFromLeaf(TreeNode* root) {
        if (!root) return "";
        stack<pair<TreeNode*, string>> st;
        st.push({root, ""});
        string ans = "";
        while (!st.empty()) {
            auto p = st.top();
            st.pop();
            if (p.first->right) {
                st.push({p.first->right, (char)(97 + p.first->val) + p.second});
            }            
            if (p.first->left) {
                st.push({p.first->left, (char)(97 + p.first->val) + p.second});
            }
            if ((p.first->left == nullptr) && (p.first->right == nullptr)) {
                string v = (char)(97 + p.first->val) + p.second;
                if ((ans == "") || (v < ans)) {
                    ans = v;   
                }                
            }
        }
        return ans;
    }
};

During the code interviews, if you are asked this question, it is better to go with the Recursion. But you can follow up with the non-recursive/iterative approach.

–EOF (The Ultimate Computing & Technology Blog) —

推荐阅读：
数学题:神舟五号飞行轨道的近地点高度为200km　 数学题:汽车在途中停了一小时，客车速度比汽车慢　 数学题:东西南北两条路交叉成直角　 数学题:哥哥和弟弟进行100米赛跑　 数学题：把14分成若干个自然数的和　 数学题:张王李赵刘5人合作完成一项工程　 数学题:姐姐8年后的年龄是妹妹3年前的5倍　 数学题:一个直角三角形以它的斜边为轴旋转一周　 数学题：一个三角形被一个长方形挡住了　 摘桃子的数学题　
评论列表
添加评论