How to Compute the Catalan Numbers using Dynamic Programming Alg
- 时间:2020-09-15 16:10:27
- 分类:网络文摘
- 阅读:103 次
Catalan numbers are popular in Combinatoric Mathematics. Many interesting counting problems tend to be solved using the Catalan numbers. The first few Catalan numbers are:
1, 1, 2, 5, 14, 42, 132, 429, 1430, 4862, 16796, 58786, 208012, 742900, 2674440, 9694845, 35357670, 129644790, 477638700, 1767263190, 6564120420, 24466267020, 91482563640, 343059613650, 1289904147324, 4861946401452
A few interesting Catalan counting problems:
- The number of different ways to cut a convex polygon into triangles i.e. n triangles – Catalan(n) ways
- The number of different ways i.e. Catalan(n) to form a full binary tree with n + 1 leaves
- The number of monotonic lattice paths (which do not pass above the diagonal) along the edges of a grid on a N x N square cells.
- Different ways to generate the parentheses: How to Generate Parentheses using Bruteforce or Depth First Search (Backtracking) Algorithms?
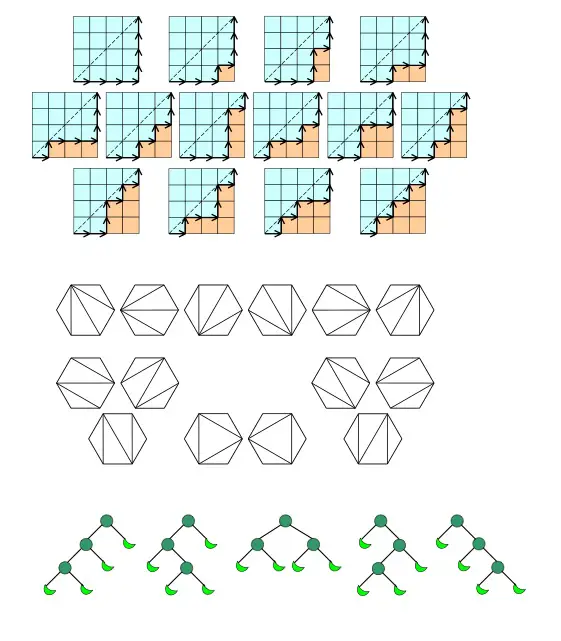
catalan-numbers-applications
Catalan Numbers Math Formula
The Catalan number can be computed in the simple form:
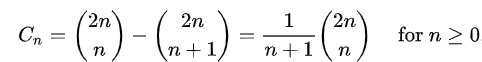
catalan-numbers-formula
There are several ways to compute the nth Catalan number. In order to compute the Catalan numbers in Dynamic Programming, we can use the following recurrence relation:

catalan-numbers-recurrence-relations
Alternatively, the following is a simple recurrence relation between Catalan number nth and nth+1.
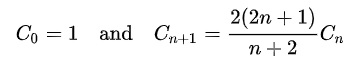
catalan-numbers-recurrence-relations-simple
See this implementation based on the above simple Catalan formula: How to Compute the Catalan Number?
How to Compute the Catalan using Recursions?
The Catalan numbers grow quickly (exponentially) and will overflow if the N is large. The following is a naive implementation of the Catalan formula.
1 2 3 4 5 6 7 8 | int64_t catlan(int n) { if (n <= 1) return 1; int res = 0; for (int i = 0; i < n; i ++) { res += catlan(i) * catlan(n - i - 1); } return res; } |
int64_t catlan(int n) {
if (n <= 1) return 1;
int res = 0;
for (int i = 0; i < n; i ++) {
res += catlan(i) * catlan(n - i - 1);
}
return res;
}However, this implementation is not practically usable as the performance complexity is exponential. The intermediate catalan numbers are computed over and over again.
Dynamic Programming: Computing Catalan Numbers using Recursion + Memoization
We can improve the above recursive implementation into Dynamic Programming by simply using a hash map to store the intermediate calculations e.g. the Memoization.
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 | unordered_map<int, int64_t> memo; int64_t catlan(int n) { if (n <= 1) return 1; if (memo.find(n) != memo.end()) { // avoid duplicate calculations return memo[n]; } int res = 0; for (int i = 0; i < n; i ++) { res += catlan(i) * catlan(n - i - 1); } memo[n] = res; // store into the memo return res; } |
unordered_map<int, int64_t> memo;
int64_t catlan(int n) {
if (n <= 1) return 1;
if (memo.find(n) != memo.end()) {
// avoid duplicate calculations
return memo[n];
}
int res = 0;
for (int i = 0; i < n; i ++) {
res += catlan(i) * catlan(n - i - 1);
}
memo[n] = res; // store into the memo
return res;
}The intermediate calculations are computed and stored into the hash map for later retrieval. The overall time complexity is N square and the space requirement is O(N).
Dynamic Programming: Computing Catalan Numbers using Iterative Approach
Requiring a O(N) vector/array to store the Catalan numbers, we can do this purely iteratively in O(N^2) time complexity. This implementation eliminates the calling stacks due to recursion and is the most efficient (in terms of time and space) among the three implementations presented in this post.
1 2 3 4 5 6 7 8 9 10 11 | int catlan(int n) { vector<int64_t> dp(n + 1, 0); dp[0] = 1; dp[1] = 1; for (int i = 2; i <= n; i ++) { for (int j = 0; j < i; ++ j) { dp[i] += dp[j] * dp[i - j - 1]; } } return (int)dp.back(); } |
int catlan(int n) {
vector<int64_t> dp(n + 1, 0);
dp[0] = 1;
dp[1] = 1;
for (int i = 2; i <= n; i ++) {
for (int j = 0; j < i; ++ j) {
dp[i] += dp[j] * dp[i - j - 1];
}
}
return (int)dp.back();
}You may be interested in this post: How to Compute the Catalan Number?
–EOF (The Ultimate Computing & Technology Blog) —
推荐阅读:就在今天,走在雪地上 元旦节的一天作文400字 恰好红叶正浓时 下雨的中秋节作文400字 我的北方,在一片雪野里生动 今天是三八妇女节作文800字 找不到一个人代替你 来吧,我是北方 什么是优角 兔子问题
- 评论列表
-
- 添加评论